The Formation of Kappa Distributions in Solar Flares

Nugget
Number:	238
1st Author:	Nicolas Bian
2nd Author:	Duncan Stackhouse
Published:	October 13, 2014
Next Nugget:	[rkeley.edu/~tohban/wiki/index.php/A_Record-Setting_CMEless_Flare Major CME-less Flare]
Previous Nugget:	Balmer continuum

Introduction

A solar flare invariably emits X-rays, and this has almost become its defining property. These X-rays come in two flavors: "hard," above about 10 keV photon energy, marking the non-thermal development of the flare; and "soft," with a well-characterized thermal spectrum including many emission lines. This latter shows thermal physics in the flare, ie with a good match to the expectation from a collisionally-dominated Maxwellian distribution function, consistent with the level populations expected from plasma temperatures in the range 10-20 x 10⁶ K. In the hard X-ray domain, understanding the particle distribution function - definitely not Maxwellian - has posed substantial theoretical problems.

In statistics, kappa distributions are best known as Student's t-distributions. In the English-language literature this 
distribution takes its name from William Sealy Gosset's 1908 paper in Biometrika under the pseudonmym "Student".
Gossett worked at the Guiness Brewery in Dublin, and was interested in the problems of small samples, for example the 
chemical properties of barley. One version of the origin of the pseudonym is that Gosset's employer preferred staff to use 
pen names when publishing scientific papers instead of their real name, therefore he used the name "Student" to hide his 
identity. Another version is that Guinness did not want their competitors to know that they were using the t-test to test 
the quality of raw material.

RHESSI has revealed a new class of hard X-ray sources in which the emission is mainly in coronal magnetic loops so dense that the accelerated electrons are stopped by collisional friction in the corona, rather than streaming through it and impacting on the chromosphere to produce hard X-ray "footpoint sources", so-called because the hard X-rays illuminate the feet of the coronal magnetic loops. Flares with HXR footpoints are common, and the new coronal sources have only been discovered recently with RHESSI. Given that the acceleration and HXR emitting regions appear to be cospatial, these flares are opening new horizons for the study of acceleration processes ( see Fig.1 ).

Fig. 1: Acceleration (yellow) and HXR (red) emission regions; (left) for the majority of flares which are dominated by HXR emission in the dense footpoints, (right) for the type of flares discovered with RHESSI which are instead dominated by emission in the dense coronal loop. But recall that with RHESSI we only see red not yellow!!!

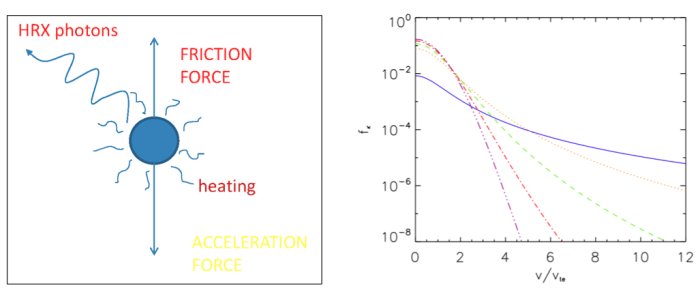
In this Nugget, we describe a model for stochastic acceleration in dense coronal loops where the velocity distribution of HXR emitting electrons is determined by a balance between acceleration and collisional friction with the dense background as drawn schematically on Fig.2.

Fig. 2: An electron accelerated in a collisional plasma emits HXR's and produces heat by collisional friction with the background. Without the acceleration vector pointing downward on this figure, this corresponds to collisional stopping of an HXR emitting electron in a dense background target with the friction force always opposite to the direction of electron motion. The situation with the acceleration vector which is random both in amplitude and direction corresponds to stochastic acceleration of an HXR emitting electron. Fig. 3: The kappa distribution f_κ, for different values of κ. For small values of κ the distribution function has a Maxwellian core and a non-thermal power-law tail. For larger values of κ, the distribution becomes almost indistinguishable from a Maxwellian (from right to left)

Such a scenario admits a stationary distribution for the electrons that takes the form of a kappa distribution with the power-law index κ. Kappa distributions have a Maxwellian-like core and a power-law tail, and they approach the standard Maxwellian distribution when κ becomes large. as illustrated in Fig. 3. Kappa distributions are now routinely used as empirical functions for the characterization of particle distribution functions in space plasmas, including electrons in solar flares (see our earlier Nugget; our new work is detailed in Ref. [1]).

An interesting thing here is that the power law index κ of the accelerated distribution of electrons is simply the ratio of the deceleration over the acceleration efficiency in this acceleration model. Interesting also, is that the power law-index κ in HXR-emitting electron energy is related to the power-law index in HXR-emitted photon energy γ by the simple relation γ=κ. Since γ is observed with the HXR-receiving instrument to be of the order of 5 in solar flares, this means that our model predicts that the deceleration efficiency of collisional friction must be an order of magnitude larger than the acceleration efficiency in the acceleration region of dense flaring coronal loops. This is one main point of this Nugget that is probably brand-new.

Finally, we show how the high-energy tails of the κ distribution are populated in this acceleration model. In fact, acceleration involves a "wave front" propagating forwards in velocity space, so that electrons of higher energy are accelerated later and on a collisional time scale τ_c. The relaxation mechanism involved in the acceleration of these electrons being in contact with the dense background is illustrated in Figure 4 showing the evolution of an initial Maxwellian thermal population of electrons (blue, solid line) toward the final kappa distribution (purple, triple dot-dash line).

Fig. 4: Temporal evolution of electron distribution function f(v,t), for κ= 5. The solid blue line shows the initial Maxwellian and then, from left to right, f(v,t) at τ_c = 1.0 (yellow dotted line), τ_c=10 (green dashed line), τ_c=100 (red dot-dashed line), and τ_c = 1000 (purple triple dot-dashed line). Fig. 5: The kappa distribution f_κ, for different values of κ. For small values of κ the distribution function has a Maxwellian core and a non-thermal power-law tail. For larger values of κ, the distribution becomes almost indistinguishable from a Maxwellian (from right to left)

In Fig.3, we plot the evolution of the normalized distribution which more clearly exhibits the "wavefront" structure moving towards higher energies.

Of course, because our model is quite simple there are a certain numbers of weaknesses associated with it. One main limitation, to our taste, is that we assume a fixed background temperature given by the observed temperature. But, given the importance of energy loss in the formation of the κ distribution of accelerated electrons in dense coronal loops, a natural problem arises whether collisional friction can also produce enough heat during the acceleration process to account for the observed loop temperatures. Given that heating through friction is a well-understood phenomenon, we have now the tools to extend this analysis to the problem of heating by electrons while being accelerated in, not outside the thick-target source. This, we hope, will soon be the subject of a future RHESSI Nugget

References

[1] "The Formation of Kappa-Distribution Accelerated Electron Populations in Solar Flares"